IMO 2023 | PROBLEM 2 - SOLUTION - 8 July 2023

Problem 2.
Let $ABC$ be an acute-angled triangle with $AB < AC$ . Let $\Omega$ be the circumcircle of $ABC$ . Let $S$ be the midpoint of the arc $CB$ of $\Omega$ containing $A$ . The perpendicular from $A$ to $BC$ meets $BS$ at $D$ and meets $\Omega$ again at $E \neq A$ . The line through $D$ parallel to $BC$ meets line $BE$ at $L$ . Denote the circumcircle of triangle $BDL$ by $\omega$ . Let $\omega$ meet $\Omega$ again at $P \neq B$ . Prove that the line tangent to $\omega$ at $P$ meets line $BS$ on the internal angle bisector of $\angle BAC$ .

proposed by Tiago Mourão and Nuno Arala, Colombia

Solution 1

$[asy] size(10cm); import olympiad; import geometry; pair A = dir(119); pair A_prime = -A; pair E = reflect((0,0), dir(90)) * A_prime; pair S = dir(90); pair D = 0.6*E + 0.4*A; pair B = 2 * foot((0,0), D, S) - S; pair P = 2 * foot((0,0), A_prime, D) - A_prime; pair Q = reflect((0,0), (P+A_prime)/2) * S; pair M = -S; pair X = extension(E,Q,S,D); pair Y = extension(P,Q,A,M); pair L = extension(D+E-A_prime, D, B,E); fill(X--M--Q--cycle, paleblue); fill(A--P--S--cycle, paleblue); draw(unitcircle, linewidth(1)); draw(S--X, red+linewidth(1.2)); draw(P--A_prime, linewidth(0.8)); draw(circle(B,D,P), linewidth(0.8)); draw(P--Q, linewidth(0.8)); draw(X--M--Q--cycle, blue+linewidth(0.8)); draw(A--P--S--cycle, blue+linewidth(0.8)); draw(A--M, linewidth(0.8)); draw(A--E--A_prime, gray+linewidth(0.7)); draw(S--M, gray+linewidth(0.7)); draw(D--L, gray+linewidth(0.7)); dot("$A$", A, dir(118)); dot("$A'$", A_prime, dir(-62)); dot("$E$", E, dir(-84)); dot("$S$", S, dir(89)); dot("$D$", D, dir(16)); dot("$B$", B, dir(-4)); dot("$P$", P, dir(133)); dot("$Q$", Q, dir(17)); dot("$M$", M, dir(-82)); dot("$X$", X, dir(-139)); dot("$Y$", Y, dir(143)); dot("$L$", L, dir(165)); [/asy]$
Let $A'$ be the antipode of $A$ in $\Omega$ . Moreover, let the tangent to $\omega$ at $P$ meet $\Omega$ again at $Q$ . Let $M$ be the other midpoint of arc $BC$ . Let $X=QE\cap MA'$ , and $Y=PQ\cap AM$ . We finish the problem in three steps.

Since $\angle BPD = \angle BLD = 90^\circ - \angle C = \angle BPA'$ , we get that $P,D,A'$ are collinear.
Pascal on $QPA'MAE$ gives $PQ\cap AM=Y$ , $PA'\cap AE = D$ , and $A'M\cap EQ = X$ are collinear.
We have
$\measuredangle APQ = 90^\circ + \measuredangle DPQ = 90^\circ + \measuredangle DBP = 90^\circ + \measuredangle SAP = \measuredangle MAP = \measuredangle MQP,$ so $AP\parallel MQ$ . Thus, $\triangle MQX$ and $\triangle APX$ are homothetic at center $AM\cap PQ=Y$ , so $S,Y,X$ are collinear.

Hence, $S, Y, D, X$ are collinear. $B$ clearly lies on this line, done.

Solution 2

Let

the intersection of the interior bisector of the angle $\angle BAC$ with

and let It $\angle PBD=x.$

be $\angle BPD=\angle BLD=\angle EBC=90^\circ-\angle C\Longrightarrow AP\perp PD.$ Additionally $\displaystyle \angle PAD=\frac{\angle A}{2}+\angle C-x.$

So $\displaystyle \frac{\sin \angle PAD}{\sin \angle PBD}=\frac{\displaystyle \sin \left ( \frac{A}{2}+B+x \right )}{\sin x}=\frac{1}{AD}\cdot \frac{BD}{\cos C}=\frac{\cos B}{\displaystyle -\cos \left ( \frac{A}{2}+B \right )\cos C}\Longleftrightarrow$

$\displaystyle \Longleftrightarrow \boxed{\cot x=\frac{\displaystyle \cos B-\cos ^{2}\left ( \frac{A}{2}+B \right )\cos \left ( A+B \right )}{\displaystyle \sin \left ( \frac{A}{2}+B \right )\cos \left ( \frac{A}{2}+B \right )\cos \left ( A+B \right )}}\left ( 1 \right )$

We it suffices to show that $RP^{2}=RD\cdot RB=RA^{2}\Longleftrightarrow RP=RA.$

It is sufficient that is $\displaystyle \frac{AP}{AR}=2\cos \angle PAR=2\cos \left ( \frac{A}{2} +C-x+90^\circ-\frac{A}{2}-C\right )=2\sin x.$

But it is true that $\displaystyle \frac{AP}{AR}=\frac{AP}{AB}\cdot \frac{AB}{AR}=\frac{\displaystyle \sin \left ( x-90^\circ+\frac{A}{2}+C \right )}{\sin \left ( A+B \right )}\cdot \frac{\displaystyle \sin \frac{A}{2}}{\displaystyle \cos \left ( \frac{A}{2}+C \right )}=\frac{\displaystyle -\cos \left ( \frac{A}{2}+B-x \right )\sin \frac{A}{2}}{\displaystyle\sin \left ( A+B \right )\cos \left ( \frac{A}{2}+B \right ) }.$

So finally it is sufficient $\displaystyle \frac{\displaystyle -\cos \left ( \frac{A}{2} +B-x\right )\sin \frac{A}{2}}{\displaystyle \sin \left ( A+B \right )\cos \left ( \frac{A}{2} +B\right )}=2\sin x\Longleftrightarrow \frac{\displaystyle -\cos \left ( \frac{A}{2}+B-x \right )}{\sin x}=\frac{\displaystyle 2\sin \left ( A+B \right )\cos \left ( \frac{A}{2}+B \right )}{\displaystyle \sin \frac{A}{2}}\Longleftrightarrow$

$\displaystyle \Longleftrightarrow \cos \left ( \frac{A}{2}+B \right )\cot x+\sin \left ( \frac{A}{2}+B \right )=\frac{\displaystyle -2\sin \left ( A+B \right )\cos \left ( \frac{A}{2}+B \right )}{\displaystyle \sin \frac{A}{2}}\Longleftrightarrow$

$\Longleftrightarrow \boxed{\cot x=\frac{\displaystyle -2\sin \left ( A+B \right )\cos \left ( \frac{A}{2}+B \right )-\sin \frac{A}{2}\sin \left ( \frac{A}{2}+B \right )}{\displaystyle \sin \frac{A}{2}\cos \left ( \frac{A}{2}+B \right )}}\left ( 2 \right )$

Therefore from 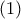$\left ( 1 \right )$ and $\left ( 2 \right )$ it is sufficient n.d.o:

$\displaystyle \frac{\displaystyle \cos B-\cos ^{2}\left ( \frac{A}{2}+B \right )\cos \left ( A+B \right )}{\displaystyle \sin \left ( \frac{A}{2}+B \right )\cos \left ( A+B \right )}=\frac{\displaystyle -2\sin \left ( A+B \right )\cos \left ( \frac{A}{2}+B \right )-\sin \frac{A}{2}\sin \left ( \frac{A}{2}+B \right )}{\displaystyle \sin \frac{A}{2}}\left ( I \right )$

which is direct. The proof is complete.